Sample Standard Deviation, PDF
-

Standard Deviation Estimator
download now -

Sample Standard Deviation and Random Sampling
download now -

Standard Deviation of Sample Means
download now -

Standard Deviation & Standard Error
download now -

Relative Standard Deviation
download now -

Standard Deviation and Variance
download now -

Standard Deviation and Confidence Limits
download now -
Simple Standard Deviation
download now -

Calculating Standard Deviation on a Calculator
download now -

What Is a Standard Deviation
download now -

Standard Deviation Ungrouped Data
download now -

Standard Deviation of a Random Variable
download now -

Standard Deviation Meta Analysis
download now -

Standard Deviation Range
download now -
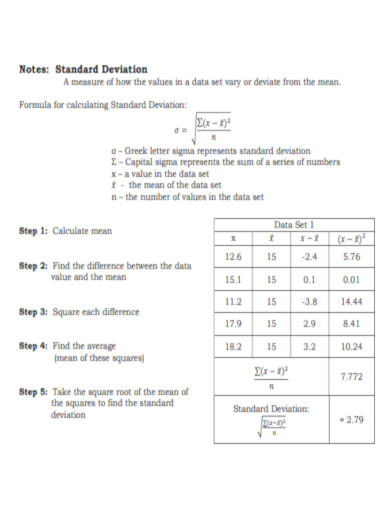
Standard Deviation Notes
download now -

Standard Deviation of a Variable in a Finite Population
download now -

Standard Deviation Comparing Means
download now -

Nature of Standard Deviation
download now -

Standard Deviation as a Measure of Risk for a Mutual Fund
download now -

Interpret Standard Deviation
download now -

Standard Deviation for charts
download now -
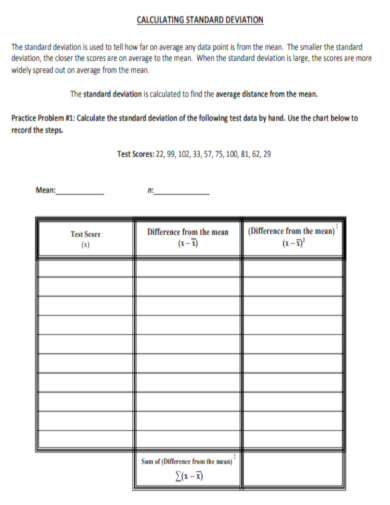
Calculating Standard Deviation
download now -

Mean and Standard Deviation
download now -
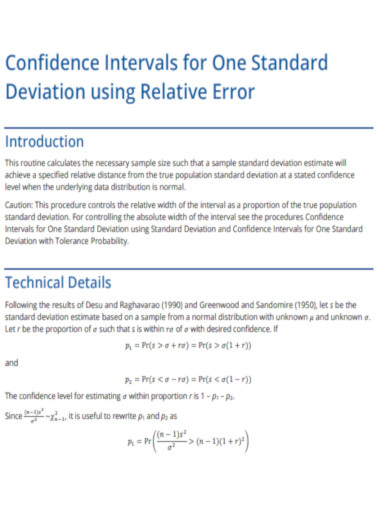
Confidence Intervals for One Standard Deviation
download now -

Detection of Drift Effects before Calculating the Standard Deviation
download now -

Standard Deviation From the Sample Size
download now -

Simulation Based Estimation of Standard Deviation for Meta-analysis
download now -

Chi-Square Test for Standard Deviation
download now -

Finding the Variance and Standard Deviation
download now -

Interpretation of Standard Deviation
download now -

Printable Standard Deviation
download now -

Use of Pooled Standard Deviation
download now -

Editable Standard Deviation
download now -

Properties of the Standard Deviation
download now -

Evolution Standard Deviation
download now -

Statistics of Standard Deviation
download now -

Basic Standard Deviation
download now -

Standard Deviation of Random Variables
download now -

Standard Deviation Accuracy
download now -

Standard Deviation Probability and Statistics
download now -

Standard Deviation Format
download now -

Standard Deviation Activity
download now -

Finding the Mean and Standard Deviation
download now -

Description of Calculating Standard Deviation
download now -

Visualizing Standard Deviation
download now -
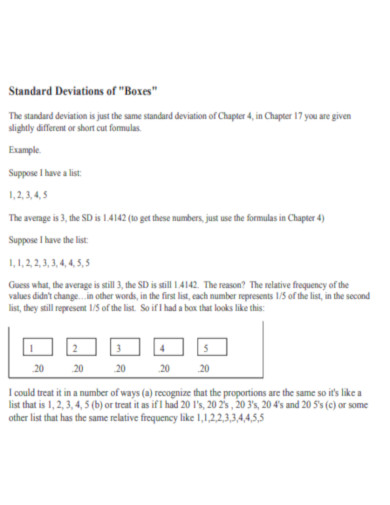
Standard Deviation of Boxes
download now -

Confidence Interval Worksheet for Standard Deviation
download now -

Standard Deviation Reported in Literature
download now -

Standard Deviation and Error Bar
download now -

Standard Deviation Steps
download now -
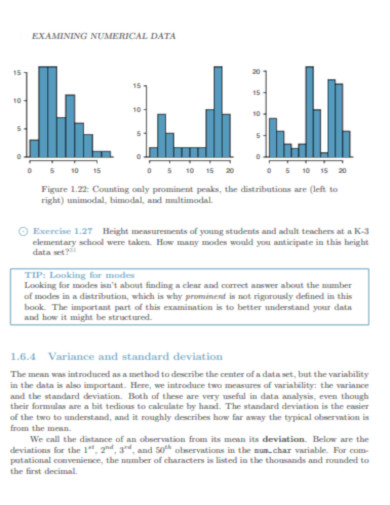
Standard Deviation Examining Numerical data
download now -

Standard Deviation Using Ti-89
download now -

Unbiased Estimation of the Standard Deviation
download now
FREE Standard Deviation s to Download
Sample Standard Deviation, PDF
What Is a Sample Standard Deviation?
Sample Standard Deviation Formula
Advantages of Sample Standard Deviation
How to Calculate Sample Standard Deviation
FAQs
What is the formula for a sample vs. population?
What is a high standard deviation?
What does the sample standard deviation tell us?
Why is a sample standard deviation important?
What is the relationship between variance and standard deviation?
What Is a Sample Standard Deviation?
A sample standard deviation is the standard measure of the variability of a sample in research. This is the square root of sample variance. The values of a data set are determined through this. In other words, this is the average data from what we are expecting. The standard deviation is small if there is little variation in the data. If the samples are the same, the sample standard variation has a small value. A sample standard deviation formula is used to calculate data. The formula has sample standard deviation symbols. You can do calculations using a sample standard deviation calculator.
It can be easy to compute sample standard deviation in Excel. But you should have an understanding of the sample standard deviation notation. Any sample standard deviation example shows how you can distribute data to the population. It can be up to you to determine the sample size from the mean and standard deviation. But all in all, the sample standard deviation makes the frequency distribution easier. There can be a confidence interval in the normal distribution. This formula can be good when we are allocating resources and doing distributions in our business processes.
Sample Standard Deviation Formula
The main formula can be the sum of squares which can get squared of deviation scores. This is worth noting to get the factors of statistical measure. Not only will you get the standard deviation, but you will also get a large degree of variability. You will know when the data is lower in value or when it is less relative. In research, sets of information are usually large. Sample standard deviation can help us to calculate everything.
So, when do we use it? Do you know how to find the sample standard deviation? Usually, the population size N is big and it is hard to get the value xi. Practically, every observation is hard to compute in variance. So, we can estimate the sample standard deviation by computing a size n from the sample. This can be taken from the data of size N. The variance that you can get is the sample standard deviation (S). These are statistics that you can get from a reference population. It is of good variability and the sample standard deviation is always greater than the population.
Sample standard deviation is one of the two types of standard deviation. The other one is population standard deviation. On the other hand, a sample proportion standard deviation is a type of sample standard deviation. To calculate this, you can use a sample proportion standard deviation calculator.
Advantages of Sample Standard Deviation
Technology and data complement each other. We use sample standard deviation because of its benefits. We get good results for our business. It is just fascinating that when we demand analysis for every sampling that we have, we can get it through the sample standard deviation. In many social media applications, we can be able to gather data so that we can serve our purpose. Now, we are going to provide you with some of the advantages of sample standard deviation. Have the following:
How to Calculate Sample Standard Deviation
Usually, a sample standard deviation is calculated automatically using a software program. This makes everything better for statistical analysis. But sometimes, you may be given the chance to compute the sample standard deviation manually. The following are the steps that you can take to calculate the sample standard deviation:
1. Calculate the Mean
The first thing that you need to do is to compute the mean. Simply, it is the average of all the numbers. You should find the average of all your samples. To do this, add all the scores of your data. Then divide the sum by the number of your data.
2. Find the Score Deviation
You should know each score’s deviation from the mean. Do this by subtracting the mean from every score. This is how you can get the deviation from the mean.
3. Square Deviations
Then, you should square each deviation from the mean. The formula is to multiply each deviation by the mean. The result should be a positive number.
4. Add the Squares
After that, you should have the sum of the squares. Add all the squared deviations. These are all the results that you have gotten.
5. Get the Variance
The sum of the squares should be divided by n-1. This is if you have to get the sample. If you have to get the population, divide the sum of the squares with N. This is referred to as the variance.
6. Find the Square Root of the Variance
The last step will be taking the square root of variance. The answer will be the standard deviation. Just follow these simple steps to get the sample standard deviation that is needed for your research.
FAQs
What is the formula for a sample vs. population?
To calculate the population standard deviation, you should divide the sample by n which is the number of values. On the other hand, the formula of sample standard deviation can be obtained by dividing n-1 which is less than the number of values.
What is a high standard deviation?
A high standard deviation is when there is a lot of variance in information regarding the mean. This means that the information is spread out well. On the contrary, a low standard deviation means that the data is clustered firmly around the mean.
What does the sample standard deviation tell us?
Sample standard deviation tells us how data is distributed. It describes each data point and gives calculated values in all proximity. Whether the data is gathered together or spread out, a sample standard deviation can tell you how far the values are from the mean.
Why is a sample standard deviation important?
A sample standard deviation can help us make a good risk assessment. Through it, we can foresee all the risks that we can have in our business. Consider an investment that you will have per year. Through sample standard deviation, you can understand the low likelihood of your ventures. You can be better at decision-making when you understand all the risks that you can have in your business.
What is the relationship between variance and standard deviation?
- The square of the standard deviation is equal to the variance.
- Variance and standard deviation have different units. The units of variance are larger than the units of standard deviation.
- Both of them give the measure of the variability of all sources.
- The average squared deviation is represented by variance while the standard deviation is the square of that number.
Whether you are having brief research or extensive research, you need to get the sample standard deviation so that you will know how to distribute everything. It will be easy for you to allocate resources. You will have the best analysis for your samples that can enable you to create the best solutions for your study.
